Here we see three identical pendulums, oscillating independently. The red and purple ones are vibrating with small amplitudes and so their periods are nearly the same. But the blue one is undergoing what would be considered to be very large amplitude oscillations and has a significantly longer period. In fact, as the amplitude approaches π radians, the period increases without bound and approaches infinity.
Recent searches
Search options
#appliedmathematics
The above is only strictly true for “small” amplitudes. If the #pendulum is subject to large amplitudes, it is no longer governed by the simple linear differential equations alluded to above. Given that the rod remains rigid and no energy is dissipated, the pendulum #equations may still be solved exactly, though they are now #nonlinear.
In elementary #mechanics we are taught about the #SimplePendulum, which is modelled as a point #mass hanging via a rigid #rod or #string of a given length, under uniform #gravity. This simple model is also useful in introducing #OrdinaryDifferentialEquations and helps us to understand #SimpleHarmonicMotion.
Job Alert
Tandem Professur (W1 LBesO) Sichere Software Systeme
Deadline: 2025-05-26
Location: Germany, Zweibrücken, Rheinland Pfalz
The analysis of artificial intelligence knowledge graphs for online music learning platform under deep learning https://www.byteseu.com/1004938/ #AI #AppliedMathematics #ArtificialIntelligence #ComputationalScience #ComputerScience #DataSparsity #FeatureFusion #HumanitiesAndSocialSciences #InformationTechnology #KnowledgeGraphs #MathematicsAndComputing #multidisciplinary #MusicRecommendationSystem #PureMathematics #Science #ScientificData #software #statistics
Job - Alert
#PhD Position (f_m_x) – Uncertainty Analysis of Coupled Reactive Transport Models
Deadline: 2025-05-27
Location: Germany, Potsdam, Brandenburg
"The "Correlation" Between Statistics and Eugenics" (2024) 2/2
https://lareviewofbooks.org/article/the-correlation-between-statistics-and-eugenics/
#statistics
#philosophy
#philosophyofscience
#mathematics
#appliedmathematics
#eugenics
@philosophy@a.gup.pe
@philosophy@newsmast.community
I cannot think of an applied mathematics that is more beautiful and far-reaching, or philosophically wilder, than probability. No, nonlinear dynamics and chaos people, it’s not even close
#probability
#mathematics
#appliedmathematics
#philosophy
#philosophyofscience
@philosophy@newsmast.community
@philosophy@a.gup.pe
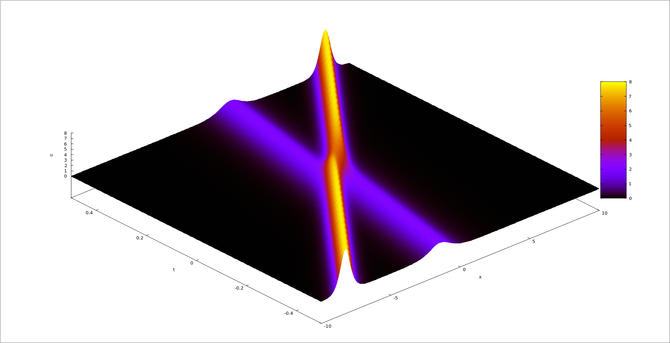
We’re having a #Soliton sale over here! Get yours quick! Seriously, though, this is a quick #animation of the #SolitonInteraction surface – the solution of the #Korteweg-deVries #Eauation showing (x,t,u(x,t)) giving you an all-round view.
I posted on #Soliton interactions in the #Korteweg-deVries #Equation yesterday with an #Animation of how two #SolitaryWaves interact. The #PhaseShift experienced by both can also be seen in a static #3D plot with time, t, plotted on one axis. You should be able to see a “dog-leg” in the trajectory of each wave after it interacts with the other.
A subtlety probably difficult to spot in the animation is that the interaction of the two waves leaves them phase shifted, with the taller wave gaining position, while the shorter one loses it. This further animation shows the interactions again (purple) but I’ve also shown what would happen if each wave moved without interaction with the other (red and blue).
Here is an example taken from Solitons: an introduction by Drazin & Johnson, where taller wave overtakes a shorter one moving in the same direction.
4/4
When you start looking at #NonlinearWaves, some of these principles no longer apply. For example, in the Korteweg-de Vries equation, which has #Soliton or solutions, you can no longer simply add two solutions together as the resulting function would not be a solution of the governing equation. Waves of different heights travel at different velocities, with the taller waves moving faster than the shorter ones. Instead, they interact #nonlinearly.
3/4
Also, waves of different heights travel at different velocities, with the taller waves moving faster than the shorter ones.
2/4
In #FluidMechanics, things like #SurfaceWaves are often modelled using #LinearEquations, which give rise to phenomena such as the principle of #LinearSuperposition, where you can take two or more distinct solutions and simply add them together to get a new solution. Solutions are also periodic and can be decomposed into sinusoidal functions.
1/4
“My PhD was all about understanding what happens to blood flow in collapsible blood vessels like the giraffe jugular vein. In my postdoc I was investigating how to optimise ventilator settings for patients in ICU and then how to deliver inhaled therapies into the lungs. Since then, my focus has been in trying to understand how diseases like Asthma and other respiratory diseases originate and then progress. This involves incorporating biology and physics into mathematical and computational models, using approaches from different areas of applied maths. More recently I have started to look into the mechanisms that could lead to a rare lung disease called lymphangioleiomyomatosis (LAM) and Long Covid.” - Bindi Brook
Job - Alert
#PHD POSITION IN SPATIO-TEMPORAL MULTI-CRITERIA DECISION ANALYSIS FOR INFORMED DECISION-MAKING 100 %
Deadline: open until filled
Location: Switzerland, Winterthur
“Since my goal was to solve a problem no one had ever solved before, it required a creative and flexible approach, one that emphasized the exploration, experimentation, and steady refinement of ideas. But perhaps the most important lesson I learned was that there is no single “correct” way to be a mathematician.” - Katy Micek
Find the full story at https://hermathsstory.eu/catherine-micek/
(Applied?) maths terminology question:
In the context of signal analysis (doing things like Fourier transforms) you might have some variable (say, t) that you're treating as being equivalent to time and you're interested in some periodic function of t (say, sin(t)) with wavelength 2pi on the t scale.
It's common to (informally?) use the term "phase" to refer to the value of t within the first cycle of the periodic function. In that usage: phase = t modulo 2pi
By extension, I have seen people refer to location anywhere on t (not just the first cycle) as "phase", but this seems to me to be ambiguous, and not necessarily helpful, terminology.
My question is: Given that the variable t is possibly something much more abstract and not necessarily "time", is there some accepted terminology for referring to the variable t in this context?
(This is possibly a non-issue for pure mathematicians because they might never talk about phase and use only symbolic representations without using descriptive words. If so, I would be seeking terminology from some applied discipline where this kind of generic descriptive labelling is used.)
In brief, if I wanted to say:
phase = x modulo 2pi
because I am talking about periodic functions of x, is there some generic descriptive term for variables like x (e.g. time-like)?
"The realization that mathematical concepts and theory could directly impact and improve real-world problems...fueled my passion for pursuing further research and applications that bridge theory with practice" - Anna Ma